Prepare for University Studies & Career Advancement

Plasma Physics

Plasma Physics

Plasma physics is the study of plasma, the fourth state of matter, which is composed of free-moving charged particles—electrons and ions—that interact through long-range electromagnetic forces. Unlike solids, liquids, and gases, plasmas exhibit collective behavior that leads to unique properties such as high electrical conductivity, dynamic responses to magnetic and electric fields, and complex wave-particle interactions. These characteristics arise from the fact that the charged particles in plasma are not bound to specific positions, allowing them to move freely and interact in ways that create both order and chaos within the medium.

Plasmas are the most abundant form of visible matter in the universe, comprising more than 99% of the observable matter. They are naturally present in stars, including our Sun, where nuclear fusion processes take place, and in phenomena such as lightning and auroras, which showcase the interplay of plasma and Earth’s magnetic field. On Earth, plasmas are utilized in a variety of applications, from the illumination provided by fluorescent lamps and plasma televisions to the sophisticated control of plasma in experimental devices. The study of plasma physics, therefore, not only deepens our understanding of cosmic processes but also paves the way for practical technologies that harness the unique properties of this state of matter.

Advancements in plasma physics are critical for several cutting-edge technologies and future energy solutions. One of the most promising applications is in nuclear fusion, where controlled plasma reactions could provide a virtually limitless and clean energy source. In addition, plasma-based technologies are integral to the development of advanced space propulsion systems, potentially enabling faster and more efficient space travel. Moreover, plasma processing is essential in semiconductor manufacturing, where plasma etching and deposition techniques are used to create intricate patterns on microchips. Together, these applications highlight the vast impact of plasma physics on both our understanding of the universe and the advancement of modern technology.

The image illustrates Plasma Physics, showcasing swirling, high-energy plasma streams influenced by electromagnetic fields, with dynamic interactions resembling cosmic and nuclear fusion processes.
The image illustrates Plasma Physics, showcasing swirling, high-energy plasma streams influenced by electromagnetic fields, with dynamic interactions resembling cosmic and nuclear fusion processes.

Characteristics of Plasma

1. Ionization

Plasmas are formed when a gas is energized to the point that electrons are stripped from atoms, creating a mixture of free electrons and positively charged ions. This process is called ionization.

  • Fully ionized plasma: Most atoms are ionized (e.g., the core of stars).
  • Partially ionized plasma: Some neutral atoms remain (e.g., Earth’s ionosphere).

2. Quasineutrality

Plasma is quasineutral, meaning the densities of positive and negative charges are nearly equal, maintaining an overall charge balance:

ne≈nin_e \approx n_i

electron density≈ion density

3. Collective Behavior

Plasma particles interact through long-range electromagnetic forces, causing collective behavior where particles respond to the averaged fields rather than individual interactions.

4. Debye Shielding

Charged particles in plasma rearrange themselves to shield out electric potentials over a characteristic distance called the Debye length

\lambda_D

λD=ε0kBTenee2

Debye length

λD

= Square Root of [{(Permittivity of Free Space)(Boltzmann Constant)(Electron Temperature)}/{(electron density)(Square of elementary charge)}]

Types of Plasma

1. Natural Plasma

  • Astrophysical Plasma: Found in stars, interstellar medium, and solar winds.
  • Ionospheric Plasma: Exists in Earth’s upper atmosphere, affecting radio communications.
  • Auroras and Lightning: Examples of atmospheric plasma on Earth.

2. Laboratory Plasma

  • Thermal Plasma: Electrons and ions are in thermal equilibrium (e.g., fusion reactors).
  • Non-Thermal Plasma: Electrons are much hotter than ions (e.g., fluorescent lamps).

3. High-Temperature Plasma

Produced in devices like tokamaks and stellarators for nuclear fusion, reaching millions of degrees Celsius.

4. Low-Temperature Plasma

Used in industrial applications, such as plasma etching and surface treatment.

Fundamental Plasma Parameters

1. Plasma Frequency ( ωp\omega_p

 

)

The natural frequency of electron oscillations in plasma:

ωp=nee2ε0me\omega_p = \sqrt{\frac{n_e e^2}{\varepsilon_0 m_e}}

Where

mem_e

is the electron mass.

2. Cyclotron Frequency ( ωc\omega_c

 

)

The frequency at which charged particles spiral around magnetic field lines:

ωc=qBm\omega_c = \frac{qB}{m}

Where

BB

is the magnetic field strength.

3. Magnetization

A plasma is said to be magnetized if the Larmor radius (radius of particle spiraling) is much smaller than the system size.

4. Plasma Beta ( β\beta

 

)

The ratio of plasma pressure to magnetic pressure:

β=nkBTB2/2μ0\beta = \frac{n k_B T}{B^2 / 2\mu_0}

  • High-beta plasmas: Pressure-dominated (fusion reactors).
  • Low-beta plasmas: Magnetically dominated (solar corona).

Basic Plasma Equations

1. Fluid Description of Plasma

Plasma behavior can be described using fluid equations, derived from Navier-Stokes and Maxwell’s equations.

Continuity Equation (Conservation of Mass):

∂n∂t+∇⋅(nv⃗)=0\frac{\partial n}{\partial t} + \nabla \cdot (n \vec{v}) = 0

Momentum Equation (Force Balance):

mn(∂v⃗∂t+(v⃗⋅∇)v⃗)=qn(E⃗+v⃗×B⃗)−∇pmn \left( \frac{\partial \vec{v}}{\partial t} + (\vec{v} \cdot \nabla) \vec{v} \right) = qn (\vec{E} + \vec{v} \times \vec{B}) – \nabla p

Energy Equation: Governs energy transport in the plasma.

2. Kinetic Description

Involves the Vlasov equation, which describes how the distribution function

f(r⃗,v⃗,t)f(\vec{r}, \vec{v}, t)

 of particles evolves over time.

∂f∂t+v⃗⋅∇f+qm(E⃗+v⃗×B⃗)⋅∇vf=0\frac{\partial f}{\partial t} + \vec{v} \cdot \nabla f + \frac{q}{m} (\vec{E} + \vec{v} \times \vec{B}) \cdot \nabla_v f = 0

Plasma Waves and Instabilities

1. Plasma Oscillations

Electrons oscillate collectively against a stationary background of ions at the plasma frequency.

2. Alfven Waves

Low-frequency oscillations that propagate along magnetic field lines, significant in space plasmas.

3. Magnetohydrodynamic (MHD) Waves

Waves in magnetized plasma that combine fluid motion with electromagnetic fields.

4. Plasma Instabilities

Disturbances that grow over time, leading to turbulence or disruptions in plasma.

  • Rayleigh-Taylor Instability: Occurs when a heavier fluid pushes against a lighter one in a gravitational field.
  • Kink Instability: Affects magnetically confined plasma in fusion reactors.

Applications of Plasma Physics

1. Controlled Nuclear Fusion

Devices like tokamaks and stellarators aim to harness the energy of fusion by confining plasma at extremely high temperatures and pressures.

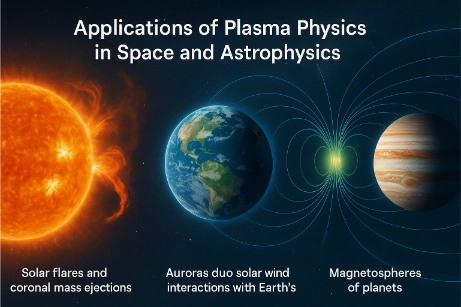
2. Space and Astrophysics

Plasma physics explains:

  • Solar flares and coronal mass ejections (CMEs).
  • Auroras due to solar wind interactions with Earth’s magnetosphere.
  • Magnetospheres of planets.

3. Plasma Propulsion

Ion thrusters and Hall-effect thrusters use plasma to propel spacecraft, offering high efficiency for deep-space missions.

4. Industrial Applications

  • Semiconductor manufacturing: Plasma etching and deposition.
  • Plasma cutting: For precision metal cutting.
  • Surface modification: Enhancing materials’ durability and conductivity.

5. Medical Applications

  • Plasma sterilization: Killing bacteria without heat.
  • Plasma medicine: Accelerating wound healing and cancer treatment.

Five Numerical Examples

Example 1: Plasma Frequency

Problem:
Find the plasma frequency for an electron density of

ne=1018 m−3n_e = 10^{18} \, \text{m}^{-3}

.

Solution:

ωp=nee2ε0me\omega_p = \sqrt{\frac{n_e e^2}{\varepsilon_0 m_e}}

ωp≈5.64×1010 rad/s\omega_p \approx 5.64 \times 10^{10} \, \text{rad/s}

Example 2: Debye Length

Problem:
Calculate the Debye length for a plasma with

Te=104 KT_e = 10^4 \, \text{K}

and

ne=1018 m−3n_e = 10^{18} \, \text{m}^{-3}

.

Solution:

λD=ε0kBTenee2≈7.43×10−5 m\lambda_D = \sqrt{\frac{\varepsilon_0 k_B T_e}{n_e e^2}} \approx 7.43 \times 10^{-5} \, \text{m}

Conclusion

Plasma physics is a rich and expansive field that explains the behavior of the most abundant state of matter in the universe. From the fusion energy that powers the stars to cutting-edge technologies like plasma propulsion and semiconductor manufacturing, the study of plasmas continues to unlock the potential for scientific and technological breakthroughs.

Review Questions and Answers:

1. What is plasma and how does it differ from the other states of matter?
Answer: Plasma is an ionized gas consisting of free electrons and ions, making it electrically conductive and highly responsive to electromagnetic fields. Unlike solids, liquids, or gases, plasma exhibits collective behavior and unique properties such as Debye shielding.

2. How is the ionization of a gas achieved to form plasma?
Answer: Ionization occurs when sufficient energy (thermal, electrical, or radiative) is supplied to a gas, freeing electrons from atoms or molecules. This process creates a mix of charged particles that defines the plasma state.

3. What is the Debye length in plasma physics, and why is it significant?
Answer: The Debye length is the scale over which electric fields are screened out in a plasma due to the redistribution of charges. It determines the distance over which charge imbalances can persist and is crucial for defining the quasi-neutrality of plasma.

4. What is plasma frequency, and what does it indicate about a plasma?
Answer: Plasma frequency is the natural oscillation frequency of electrons in a plasma when disturbed from equilibrium. It indicates how fast the plasma can respond to electric field changes and plays a key role in wave propagation and shielding.

5. How do electromagnetic fields influence the behavior of plasmas?
Answer: In plasma, electromagnetic fields exert forces on the charged particles, driving complex motions such as gyration, drift, and wave propagation. These interactions are described by magnetohydrodynamics and are central to many plasma applications.

6. What role does temperature play in determining plasma properties?
Answer: Temperature in plasma governs the kinetic energy of particles, affecting ionization levels, collision rates, and conductivity. High temperatures generally lead to higher degrees of ionization and more dynamic behavior.

7. How is plasma used in controlled nuclear fusion experiments?
Answer: In fusion experiments, plasma is confined using magnetic fields (magnetic confinement) or inertial methods to achieve conditions necessary for fusion reactions. The aim is to produce energy by fusing light nuclei, such as deuterium and tritium, under extreme temperatures and pressures.

8. What are some common instabilities observed in plasmas, and why are they important?
Answer: Instabilities such as the Rayleigh–Taylor, Kelvin–Helmholtz, and kink instabilities can disrupt plasma confinement. Understanding and controlling these instabilities are critical for achieving stable plasma in fusion reactors and other applications.

9. How do laboratory plasmas differ from astrophysical plasmas?
Answer: Laboratory plasmas are typically created under controlled conditions and may have higher densities and lower temperatures compared to astrophysical plasmas, which exist naturally in vast, diffuse regions like the solar wind or interstellar medium and often exhibit complex magnetic field structures.

10. What measurement techniques are used to diagnose plasma properties in experiments?
Answer: Diagnostic techniques include Langmuir probes, interferometry, spectroscopy, and magnetic probes. These methods help determine parameters such as electron density, temperature, and magnetic field strength, which are vital for understanding plasma behavior.

Thought-Provoking Questions and Answers:

1. How does the concept of Debye shielding influence the behavior of plasmas in both laboratory and astrophysical settings?
Answer: Debye shielding prevents long-range electric fields from influencing plasma behavior by rapidly neutralizing charge imbalances. In laboratories, it ensures that plasmas remain quasi-neutral, while in astrophysical environments, it affects phenomena like star formation and the propagation of charged particles in space.

2. In what ways could breakthroughs in plasma confinement lead to practical fusion energy?
Answer: Improved plasma confinement, such as through advanced magnetic configurations or inertial confinement techniques, can reduce energy losses and sustain the high temperatures and pressures required for fusion. This would make fusion energy a viable, clean power source, transforming global energy production.

3. How might the study of plasma instabilities contribute to our understanding of space weather phenomena?
Answer: Plasma instabilities are key drivers of solar flares, coronal mass ejections, and other space weather events. By studying these instabilities, scientists can better predict space weather, protect satellites and power grids, and understand the fundamental processes occurring in the Sun’s atmosphere.

4. What potential do nanostructured materials have in manipulating plasma behavior for advanced applications?
Answer: Nanostructured materials can offer tailored electromagnetic properties and enhanced surface interactions, which may be used to control plasma boundaries, enhance confinement, or improve energy absorption. This could lead to more efficient fusion reactors, plasma-based sensors, and novel propulsion systems.

5. How do differences in plasma density and temperature affect the design of plasma diagnostic tools?
Answer: Diagnostic tools must be sensitive enough to measure the high-speed, low-density, or high-temperature conditions typical in different plasmas. Tailoring instruments like Langmuir probes or spectrometers to specific plasma parameters is crucial for accurate measurement and analysis.

6. How can advances in computational simulations deepen our understanding of magnetohydrodynamic (MHD) phenomena in plasmas?
Answer: High-fidelity simulations allow for the modeling of complex plasma behavior under varying conditions, capturing turbulence, instabilities, and non-linear interactions. These computational tools enable researchers to test theories, optimize fusion reactor designs, and predict plasma responses in space weather.

7. In what ways might plasma physics contribute to the development of next-generation propulsion systems for space exploration?
Answer: Plasma propulsion systems, such as ion thrusters and Hall-effect thrusters, leverage plasma properties to produce high-efficiency, low-thrust propulsion. Advances in plasma control and energy management could result in more efficient spacecraft propulsion, enabling longer missions and deeper space exploration.

8. How do electromagnetic waves interact with plasmas, and what implications does this have for communication technologies in space?
Answer: Electromagnetic waves can be absorbed, reflected, or refracted by plasmas, influencing signal propagation in space. Understanding these interactions is crucial for designing communication systems that can withstand space weather effects and maintain reliable contact with spacecraft.

9. How might the integration of plasma physics with renewable energy technologies impact the future of power generation?
Answer: Plasma processes can be used to enhance energy conversion in solar cells and to develop plasma-based chemical reactors for fuel production. Integrating plasma physics with renewable energy can lead to more efficient, sustainable, and versatile power generation methods.

10. What are the challenges and prospects of using plasmas for environmental applications, such as waste treatment or water purification?
Answer: Plasmas can generate reactive species that break down pollutants and sterilize water. Challenges include controlling plasma reactions, scaling up the technology, and ensuring energy efficiency. Advances in plasma technology could lead to effective, eco-friendly solutions for environmental remediation.

11. How does the interplay between magnetic fields and plasma turbulence affect the stability of fusion reactors?
Answer: Turbulence in plasma can lead to enhanced transport of energy and particles, undermining confinement in fusion reactors. Understanding and mitigating turbulence through magnetic field configuration and plasma control strategies is vital for achieving stable, sustained fusion reactions.

12. How might future interdisciplinary research in plasma physics and materials science lead to breakthroughs in energy storage and conversion?
Answer: Interdisciplinary research can develop new materials that withstand extreme plasma conditions while efficiently converting energy. Such breakthroughs could enhance the performance of plasma-based energy systems, lead to innovative energy storage solutions, and open up new avenues in both renewable energy and fusion power.

Numerical Problems and Solutions:

1. A plasma has an electron density of 1×10181 \times 10^{18} m−3^{-3} and an electron temperature of 10 eV. Calculate the Debye length.
Solution:
  Convert temperature: T=10 eV=10×1.602×10−19 J=1.602×10−18 JT = 10 \, \text{eV} = 10 \times 1.602 \times 10^{-19} \, \text{J} = 1.602 \times 10^{-18} \, \text{J}
  Debye length, λD=ε0kBTnee2\lambda_D = \sqrt{\frac{\varepsilon_0 k_B T}{n_e e^2}}.
  Using ε0=8.85×10−12 F/m \varepsilon_0 = 8.85 \times 10^{-12} \, \text{F/m}, kB=1.38×10−23 J/Kk_B = 1.38 \times 10^{-23} \, \text{J/K} (but note we already converted T to Joules), and e=1.602×10−19 Ce = 1.602 \times 10^{-19} \, \text{C}.
  A more direct formula (in SI) is:
  λD=ε0Tnee2\lambda_D = \sqrt{\frac{\varepsilon_0 T}{n_e e^2}}
  λD=8.85×10−12×1.602×10−181×1018×(1.602×10−19)2\lambda_D = \sqrt{\frac{8.85 \times 10^{-12} \times 1.602 \times 10^{-18}}{1 \times 10^{18} \times (1.602 \times 10^{-19})^2}}
  Calculate denominator: (1.602×10−19)2≈2.566×10−38(1.602 \times 10^{-19})^2 \approx 2.566 \times 10^{-38}
  Then, numerator: 8.85×10−12×1.602×10−18≈1.418×10−298.85 \times 10^{-12} \times 1.602 \times 10^{-18} \approx 1.418 \times 10^{-29}.
  Thus, λD=1.418×10−291×1018×2.566×10−38=1.418×10−292.566×10−20\lambda_D = \sqrt{\frac{1.418 \times 10^{-29}}{1 \times 10^{18} \times 2.566 \times 10^{-38}}} = \sqrt{\frac{1.418 \times 10^{-29}}{2.566 \times 10^{-20}}}.
  =5.528×10−10≈2.35×10−5 m= \sqrt{5.528 \times 10^{-10}} \approx 2.35 \times 10^{-5} \, \text{m}.

2. Calculate the electron plasma frequency for a plasma with electron density 5×10185 \times 10^{18} m−3^{-3}.
Solution:
  Plasma frequency, ωpe=nee2ε0me\omega_{pe} = \sqrt{\frac{n_e e^2}{\varepsilon_0 m_e}}.
  Using ne=5×1018 m−3 n_e = 5 \times 10^{18} \, \text{m}^{-3}, e=1.602×10−19 C e = 1.602 \times 10^{-19}\, \text{C}

ε0=8.85×10−12 F/m\varepsilon_0 = 8.85 \times 10^{-12}\, \text{F/m}, me=9.11×10−31 kg m_e = 9.11 \times 10^{-31}\, \text{kg}.
  ωpe=5×1018×(1.602×10−19)28.85×10−12×9.11×10−31\omega_{pe} = \sqrt{\frac{5 \times 10^{18} \times (1.602 \times 10^{-19})^2}{8.85 \times 10^{-12} \times 9.11 \times 10^{-31}}}.
  (1.602×10−19)2≈2.566×10−38 (1.602 \times 10^{-19})^2 \approx 2.566 \times 10^{-38}.
  Numerator = 5×1018×2.566×10−38=1.283×10−195 \times 10^{18} \times 2.566 \times 10^{-38} = 1.283 \times 10^{-19}.
  Denom = 8.85×10−12×9.11×10−31≈8.064×10−428.85 \times 10^{-12} \times 9.11 \times 10^{-31} \approx 8.064 \times 10^{-42}
  ωpe=1.283×10−198.064×10−42=1.59×1022≈1.26×1011 rad/s\omega_{pe} = \sqrt{\frac{1.283 \times 10^{-19}}{8.064 \times 10^{-42}}} = \sqrt{1.59 \times 10^{22}} \approx 1.26 \times 10^{11} \, \text{rad/s}.

3. A plasma has a magnetic field of 0.05 T. Calculate the electron cyclotron frequency.
Solution:
  Cyclotron frequency, ωce=eBme\omega_{ce} = \frac{eB}{m_e}.
  ωce=1.602×10−19×0.059.11×10−31≈8.01×10−219.11×10−31≈8.79×109 rad/s \omega_{ce} = \frac{1.602 \times 10^{-19} \times 0.05}{9.11 \times 10^{-31}} \approx \frac{8.01 \times 10^{-21}}{9.11 \times 10^{-31}} \approx 8.79 \times 10^{9} \, \text{rad/s}

4. In a fusion reactor, the plasma temperature is 15 keV. Convert this temperature to Kelvin.
Solution:
  1 eV≈11600 K1 \, \text{eV} \approx 11600 \, \text{K}, so 15 keV=15,000 eV15 \, \text{keV} = 15,000 \, \text{eV}.
  Temperature T≈15,000×11600=1.74×108 KT \approx 15,000 \times 11600 = 1.74 \times 10^{8} \, \text{K}.

5. A magnetically confined plasma has an electron density of 1×10201 \times 10^{20} m−3^{-3}. Calculate its Debye length if the electron temperature is 100 eV.
Solution:
  T=100 eV=100×1.602×10−19T = 100 \, \text{eV} = 100 \times 1.602 \times 10^{-19} \, \text{J} = 1.602 \times 10^{-17} \, \text{J}.
  λD=ε0Tnee2=8.85×10−12×1.602×10−171×1020×(1.602×10−19)2\lambda_D = \sqrt{\frac{\varepsilon_0 T}{n_e e^2}} = \sqrt{\frac{8.85 \times 10^{-12} \times 1.602 \times 10^{-17}}{1 \times 10^{20} \times (1.602 \times 10^{-19})^2}}.
  ≈2.566×10−38 (1.602 \times 10^{-19})^2 \approx 2.566 \times 10^{-38}.
  Numerator = 8.85×10−12×1.602×10−17≈1.417×10−288.85 \times 10^{-12} \times 1.602 \times 10^{-17} \approx 1.417 \times 10^{-28}.
  Denom = 1×1020×2.566×10−38=2.566×10−181 \times 10^{20} \times 2.566 \times 10^{-38} = 2.566 \times 10^{-18}.
  λD=1.417×10−282.566×10−18≈5.523×10−11≈7.43×10−6 m\lambda_D = \sqrt{\frac{1.417 \times 10^{-28}}{2.566 \times 10^{-18}}} \approx \sqrt{5.523 \times 10^{-11}} \approx 7.43 \times 10^{-6} \, \text{m}.

6. Calculate the energy (in joules) of a photon with a wavelength of 500 nm.
Solution:
  Energy, E=hcλE = \frac{hc}{\lambda}, where h=6.626×10−34 J/s  and c=3.00×108 m/sc = 3.00 \times 10^8 \, \text{m/s}.
  λ=500 nm=500×10−9 m\lambda = 500 \, \text{nm} = 500 \times 10^{-9} \, \text{m}.
  E=6.626×10−34×3.00×108500×10−9≈1.9878×10−255×10−7≈3.976×10−19 JE = \frac{6.626 \times 10^{-34} \times 3.00 \times 10^8}{500 \times 10^{-9}} \approx \frac{1.9878 \times 10^{-25}}{5 \times 10^{-7}} \approx 3.976 \times 10^{-19} \, \text{J}

7. A plasma in a laboratory experiment has an electron plasma frequency of 2×10112 \times 10^{11} rad/s. Estimate the electron density in the plasma.
Solution:
  ωpe=nee2ε0me\omega_{pe} = \sqrt{\frac{n_e e^2}{\varepsilon_0 m_e}}
  Squaring both sides: ne=ε0meωpe2e2n_e = \frac{\varepsilon_0 m_e \omega_{pe}^2}{e^2}.
  ne=8.85×10−12×9.11×10−31×(2×1011)2(1.602×10−19)2 n_e = \frac{8.85 \times 10^{-12} \times 9.11 \times 10^{-31} \times (2 \times 10^{11})^2}{(1.602 \times 10^{-19})^2}.
  (2×1011)2=4×1022 (2 \times 10^{11})^2 = 4 \times 10^{22}.
  Numerator = 8.85×10−12×9.11×10−31×4×1022≈3.23×10−208.85 \times 10^{-12} \times 9.11 \times 10^{-31} \times 4 \times 10^{22} \approx 3.23 \times 10^{-20}.
  Denom = (1.602×10−19)2≈2.566×10−38(1.602 \times 10^{-19})^2 \approx 2.566 \times 10^{-38}.
  ne≈3.23×10−202.566×10−38≈1.26×1018 m−3 n_e \approx \frac{3.23 \times 10^{-20}}{2.566 \times 10^{-38}} \approx 1.26 \times 10^{18} \, \text{m}^{-3}.

8. A magnetic field of 0.1 T is applied to a plasma. Calculate the electron cyclotron frequency.
Solution:
  ωce=eBme=1.602×10−19×0.19.11×10−31≈1.602×10−209.11×10−31≈1.758×1010 rad/s \omega_{ce} = \frac{eB}{m_e} = \frac{1.602 \times 10^{-19} \times 0.1}{9.11 \times 10^{-31}} \approx \frac{1.602 \times 10^{-20}}{9.11 \times 10^{-31}} \approx 1.758 \times 10^{10} \, \text{rad/s}.

9. In a tokamak, the plasma current is 1 MA (1×10⁶ A) and flows through a cross-sectional area of 5 m². Estimate the current density in the plasma.
Solution:
  Current density, J=IA=1×106 A5 m2=2×105 A/m2J = \frac{I}{A} = \frac{1 \times 10^6 \, \text{A}}{5 \, \text{m}^2} = 2 \times 10^5 \, \text{A/m}^2.

10. A plasma has an electron temperature of 50 eV. Convert this temperature to joules.
Solution:
  1 eV=1.602×10−19 J1 \, \text{eV} = 1.602 \times 10^{-19} \, \text{J}.
  50 eV=50×1.602×10−19 J=8.01×10−18 J50 \, \text{eV} = 50 \times 1.602 \times 10^{-19} \, \text{J} = 8.01 \times 10^{-18} \, \text{J}.

11. A Langmuir probe in a plasma measures an electron saturation current of 2 mA. If the probe area is 1×10−61 \times 10^{-6} m² and the electron temperature is 10 eV, estimate the electron density using a simplified formula Ies≈0.6 e ne AkBTemeI_{es} \approx 0.6\,e\,n_e\,A\sqrt{\frac{k_B T_e}{m_e}}.
Solution:
  Given Ies=2×10−3 AI_{es} = 2 \times 10^{-3}\, \text{A}, A=1×10−6 m2A = 1 \times 10^{-6}\, \text{m}^2, Te=10 eVT_e = 10\, \text{eV}.
  Convert TeT_e to Joules: Te=10×1.602×10−19=1.602×10−18 JT_e = 10 \times 1.602 \times 10^{-19} = 1.602 \times 10^{-18}\, \text{J}.
  kB=1.38×10−23 J/Kk_B = 1.38 \times 10^{-23}\, \text{J/K} is not needed since we already have energy in joules, so assume TeT_e in joules is used directly.
  me=9.11×10−31 kgm_e = 9.11 \times 10^{-31}\, \text{kg}.
  Rearrange:
  ne≈Ies0.6 e ATemen_e \approx \frac{I_{es}}{0.6\,e\,A \sqrt{\frac{T_e}{m_e}}}.
  Compute Teme=1.602×10−189.11×10−31≈1.757×1012≈1.325×106 m/s\sqrt{\frac{T_e}{m_e}} = \sqrt{\frac{1.602 \times 10^{-18}}{9.11 \times 10^{-31}}} \approx \sqrt{1.757 \times 10^{12}} \approx 1.325 \times 10^6 \, \text{m/s}.
  Now,
  ne≈2×10−30.6×1.602×10−19×1×10−6×1.325×106n_e \approx \frac{2 \times 10^{-3}}{0.6 \times 1.602 \times 10^{-19} \times 1 \times 10^{-6} \times 1.325 \times 10^6}.
  Denom = 0.6×1.602×10−19×1×10−6×1.325×106≈1.275×10−190.6 \times 1.602 \times 10^{-19} \times 1 \times 10^{-6} \times 1.325 \times 10^6 \approx 1.275 \times 10^{-19}.
  Thus, ne≈2×10−31.275×10−19≈1.57×1016 m−3n_e \approx \frac{2 \times 10^{-3}}{1.275 \times 10^{-19}} \approx 1.57 \times 10^{16} \, \text{m}^{-3}.

12. In a plasma experiment, a coil with 50 turns and an area of 0.002 m² is used to measure a changing magnetic field. If the magnetic flux through the coil changes by 0.005 Wb in 0.1 s, calculate the induced EMF.
Solution:
  Total change in flux for the coil: ΔΦ_total = 0.005 Wb.
  Induced EMF, ∣ε∣=ΔΦtotalΔt=0.0050.1=0.05 V|\varepsilon| = \frac{\Delta \Phi_{\text{total}}}{\Delta t} = \frac{0.005}{0.1} = 0.05 \, \text{V}.